
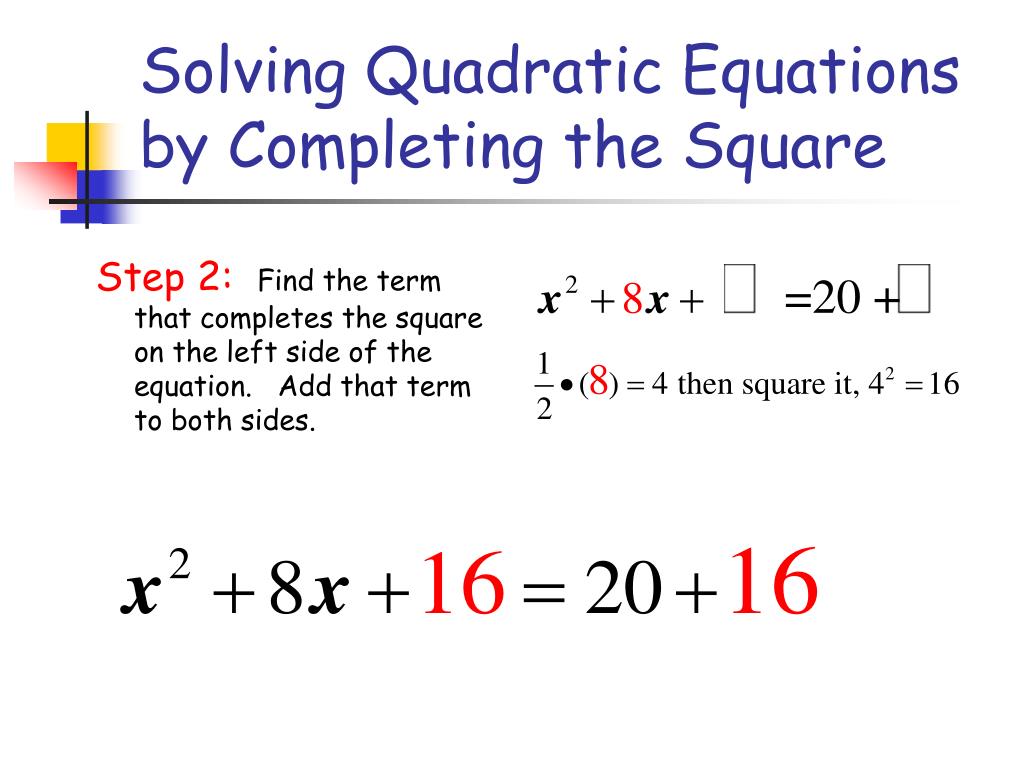
Therefore, the equation x 4 − 4 x 2 − 32 = 0 has four solutions it occurs 3 times. Since u = x 2 we can back substitute and then solve for x. (You can also use your calculator to get the solutions.) Take the two solutions and put them in factors. U 2 − 4 u − 32 = 0 ( u − 8 ) ( u + 4 ) = 0 u = 8 o r u = − 4 Use the quadratic formula to get solutions for x. In this module, we will review solving quadratic equations using factoring, using graphs, and using the square root method, as well as introduce solving quadratic equations by completing the square. This substitution transforms the equation into a familiar quadratic equation in terms of u which, in this case, can be solved by factoring. In your introductory algebra course, you should have solved quadratic equations using factoring, graphs, the quadratic formula, and the square root method. If we let u = x 2 then u 2 = ( x 2 ) 2 = x 4 and we can write For example, consider the following fourth-degree polynomial equation,

If we can express an equation in quadratic form, then we can use any of the techniques used to solve quadratic equations. In two variables, the general quadratic equation is ax2 + bxy + cy2 + dx + ey + f 0, in which a, b, c, d, e, and f are arbitrary constants and a, c 0. And, contrary to popular belief, the quadratic formula does exist outside of math class. There are other methods, like factoring or completing the square, but the quadratic formula is usually the most straightforward (and least messy) way to solve a quadratic equation. ( t + 2 t ) 2 + 8 ( t + 2 t ) + 7 = 0 ⇒ u = t + 2 t u 2 + 8 u + 7 = 0 x 2 / 3 − 3 x 1 / 3 − 10 = 0 ⇒ u = x 1 / 3 u 2 − 3 u − 10 = 0 3 y − 2 + 7 y − 1 − 6 = 0 ⇒ u = y − 1 3 u 2 + 7 u − 6 = 0 The quadratic formula, as you can imagine, is used to solve quadratic equations. Here u represents an algebraic expression. We begin by defining quadratic form An equation of the form a u 2 + b u + c = 0 where a, b and c are real numbers and u represents an algebraic expression., In this section we outline an algebraic technique that is used extensively in mathematics to transform equations into familiar forms.


 0 kommentar(er)
0 kommentar(er)
